When an idea starts to take shape
I am the Teaching and Learning Adviser for Maths and Numeracy for Jersey in the Channel Islands. Having purchased some Polydron for delivering some class based reasoning activities, I started to wonder about the possibility of running an interschool competition where the rounds of the competition are hands on construction activities with Polydron.
However, as the ideas start to mount so does the number of pieces of Polydron that are needed.
For example, how many tetrahedra can a team build in five minutes?
Teams should be made to estimate the number of triangles they would need in advance.
Well, clearly I needed to model possible timings. The clock certainly adds pressure to the usually therapeutic nature of creating models. I managed an average of about 15 secs per tetrahedron so:


Assuming teams of 4 and maybe 12 teams, the number of equilateral triangles would be:
| Teams | x | individuals | x | time (mins) | x | tetrahedron / min | x | triangles in a tetrahedron | ||
|---|---|---|---|---|---|---|---|---|---|---|
| 12 | x | 4 | x | 5 | x | 4 | x | 4 | = | 3840 triangles! |
This was going to need more Polydron than I had, even if the teams are given less time.
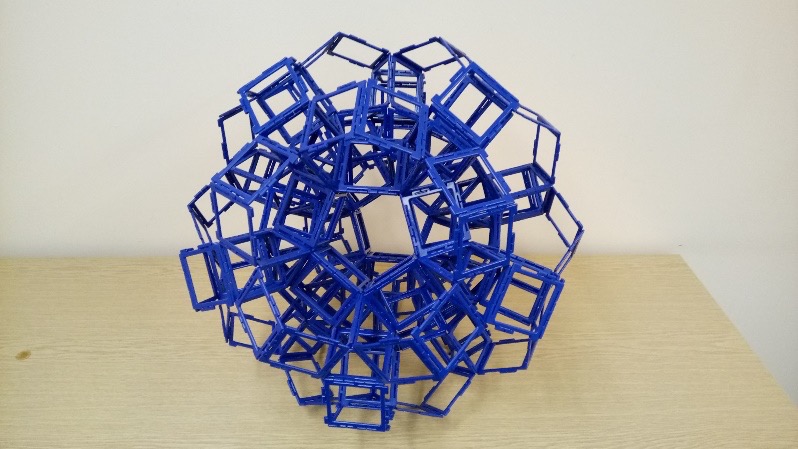
The key was that I want to create an event with an WOW factor, where pupils experience Maths on a larger scale.
I contacted Polydron with some suggestions and very quickly Polydron Director, Cyril Dowling came back to me saying, he really liked the concept and very kindly offered the practical support of more pieces.
When the box arrived, there was a very pleasant surprise. It also contained some magnetic pieces which are so tactile and a joy to use. I have already used them in demonstration talks on topics from fractions to negative numbers.

Representing 5 - -2
0 = 2 + -2 (green represents positive, red negative): definition of negative 2.
5 = 5 + 0
5 = 5 + (2 + -2)
5 = (5 + 2) + -2
So 5 - -2 = 7
They fit together so quickly and effortlessly. I hate to think how many more tetrahedrons in the time I could make with these. More importantly the ideas were also coming quickly and effortlessly and I needed to involve the local schools to create something valuable.
Schools have been very positive about the possibility of running events but feel it would be more appropriate to run say a year group competition in school initially rather than interschool version. So this is where we are at. Plans are currently being set up to run non curriculum events in school. However, activities will be explicitly mapped to the curriculum and teachers can use the activities as a method of formative assessment.
I hope that this will become a cross phase initiative which develops themes across the Key Stages and that pupils will be involved in events more frequently than a one off special day.
My intention, as with any good learning, is to journal the progress of these events and activities and to share the experiences of both the planning and reactions of pupils and staff. This is in order to develop a high class model of practical reasoning that mixes concrete and abstract mathematical ideas in a stimulating environment for pupils of all abilities. For pupils to be able to see that Mathematics can be both practical and theoretical but most importantly both aspects can be fun.
Andy Parkinson
Teaching and Learning Adviser Mathematics and Numeracy Education Department, Highlands Campus, Jersey